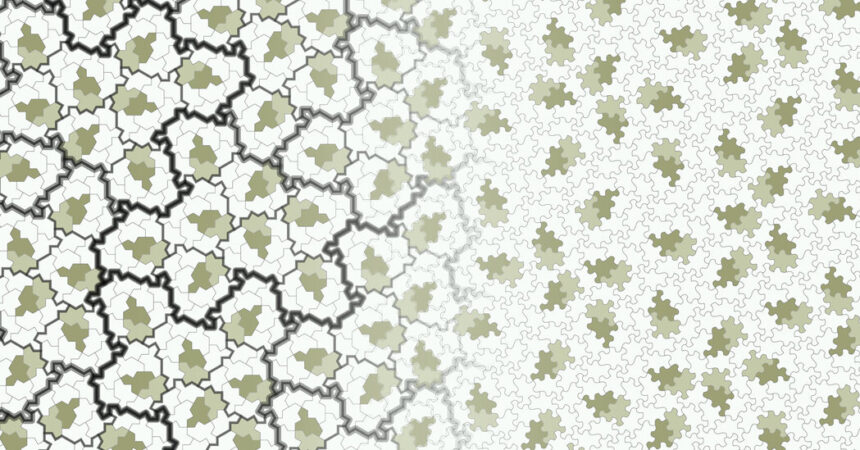
In March, a crew of mathematical tilers introduced their answer to a storied downside: That they had found an elusive “einstein” — a single form that tiles a airplane, or an infinite two-dimensional flat floor, however solely in a nonrepeating sample. “I’ve at all times wished to make a discovery,” David Smith, the form hobbyist whose authentic discover spurred the analysis, stated on the time.
Mr. Smith and his collaborators named their einstein “the hat.” (The time period “einstein” comes from the German “ein stein,” or “one stone” — extra loosely, “one tile” or “one form.”) It has since been fodder for Jimmy Kimmel, a bathe curtain, a quilt, a soccer ball and cookie cutters, amongst different doodads. Hatfest is going on on the College of Oxford in July.
“Who would imagine that slightly polygon may kick up such a fuss,” stated Marjorie Senechal, a mathematician at Smith Faculty who’s on the roster of audio system for the occasion.
The researchers may need been happy with the invention and the hullabaloo, and left nicely sufficient alone. However Mr. Smith, of Bridlington in East Yorkshire, England, and often called an “imaginative tinkerer,” couldn’t cease tinkering. Now, two months later, the crew has one-upped itself with a new-and-improved einstein. (Papers for each outcomes should not but peer reviewed.)
This tiling pursuit first started within the Sixties, when the mathematician Hao Wang conjectured that it might be unattainable to discover a set of shapes that might tile a airplane solely aperiodically. His pupil Robert Berger, now a retired electrical engineer in Lexington, Mass., proceeded to discover a set of 20,426 tiles that did so, adopted by a set of 104. By the Nineteen Seventies, Sir Roger Penrose, a mathematical physicist at Oxford, had introduced it all the way down to two.
After which got here the monotile hat. However there was a quibble.
Dr. Berger (amongst others, together with the researchers of the current papers) famous that the hat tiling makes use of reflections — it consists of each the hat-shaped tile and its mirror picture. “If you wish to be choosy about it, you’ll be able to say, nicely, that’s probably not a one-tile set, that’s a two-tile set, the place the opposite tile occurs to be a mirrored image of the primary,” Dr. Berger stated.
“To some extent, this query is about tiles as bodily objects slightly than mathematical abstractions,” the authors wrote within the new paper. “A hat reduce from paper or plastic can simply be turned over in three dimensions to acquire its reflection, however a glazed ceramic tile can not.”
The brand new monotile discovery doesn’t use reflections. And the researchers didn’t should look far to seek out it — it’s “an in depth relative of the hat,” they famous.
“I wasn’t stunned that such a tile existed,” stated the co-author Joseph Myers, a software program developer in Cambridge, England. “That one existed so carefully associated to the hat was stunning.”
Initially, the crew found that the hat was a part of a morphing continuum — an uncountable infinity of shapes, obtained by growing and lowering the perimeters of the hat — that produce aperiodic tilings utilizing reflections.
However there was an exception, a “rogue member of the continuum,” stated Craig Kaplan, a co-author and a pc scientist on the College of Waterloo. This form, technically often called Tile (1,1), could be considered an equilateral model of the hat and as such shouldn’t be an aperiodic monotile. (It generates a easy periodic tiling.) “It’s type of ridiculous and superb that that form occurs to have a hidden superpower,” Dr. Kaplan stated — a superpower that unlocked the brand new discovery.
Impressed by explorations by Yoshiaki Araki, president of the Japan Tessellation Design Affiliation in Tokyo, Mr. Smith started tinkering with Tile (1,1) shortly after the primary discovery was posted on-line in March. “I machine-cut shapes from card, to see what may occur if I had been to make use of solely unreflected tiles,” he stated in an e-mail. Mirrored tiles had been forbidden “by fiat,” because the authors put it.
Mr. Smith stated, “It wasn’t lengthy earlier than I produced a pretty big patch” — becoming tiles collectively like a jigsaw puzzle, with no overlaps or gaps. He knew he was on to one thing.
Investigating additional — with a mixture of conventional mathematical reasoning and drawing, plus computational handiwork by Dr. Kaplan and Dr. Myers — the crew proved that this tiling was certainly aperiodic.
“We name this a ‘weakly chiral aperiodic monotile,’” Dr. Kaplan defined on social media. “It’s aperiodic in a reflection-free universe, however tiles periodically should you’re allowed to make use of reflections.”
The adjective “chiral” means “handedness,” from the Greek “kheir,” for “hand.” They known as the brand new aperiodic tiling “chiral” as a result of it’s composed completely of both left- or right-handed tiles. “You may’t combine the 2,” stated Chaim Goodman-Strauss, a co-author and outreach mathematician on the Nationwide Museum of Arithmetic in New York.
The crew then went one higher: They produced a household of robust or “strictly chiral aperiodic monotiles” by means of a easy modification of the T(1,1) tile: They changed the straight edges with curves.
Named “Spectres,” these monotiles, owing to their curvy contours, solely permit nonperiodic tilings, and with out reflections. “A left-handed Spectre can not interlock with its right-handed mirror picture,” stated Dr. Kaplan.
“Now there is no such thing as a quibbling about whether or not the aperiodic tile set has one or two tiles,” Dr. Berger stated in an e-mail. “It’s satisfying to see a glazed ceramic einstein.”
Doris Schattschneider, a mathematician at Moravian College, stated, “That is extra what I’d have anticipated of an aperiodic monotile.” On a tiling listserv, she had simply seen a playful “Escherization” (after the Dutch artist M.C. Escher) of the Spectre tile by Dr. Araki, who known as it a “twinhead pig.”
“It’s not easy just like the hat,” Dr. Schattschneider stated. “This can be a actually unusual tile. It appears like a mistake of nature.”